

The mechanical properties of a material affect how it behaves as it is loaded. The elastic modulus of the material affects how much it deflects under a load, and the strength of the material determines the stresses that it can withstand before it fails. The ductility of a material also plays a significant role in determining when a material will break as it is loaded beyond its elastic limit. Because every mechanical system is subjected to loads during operation, it is important to understand how the materials that make up those mechanical systems behave.
This page describes the mechanical properties of materials that are relevant to the design and analysis of mechanical systems.
Related Pages:The relationship between stress and strain in a material is determined by subjecting a material specimen to a tension or compression test. In this test, a steadily increasing axial force is applied to a test specimen, and the deflection is measured as the load is increased. These values can be plotted as a load-deflection curve. The deflection in the test specimen is dependent on both the material's elastic modulus as well as the geometry of the specimen (area and length). Since we are interested material behavior without regard to geometry, it is useful to generalize the data to remove the effect of geometry. This is done by converting the load values to stress values and converting the deflection values to strain values:
In the equation for stress, P is the load and A0 is the original cross-sectional area of the test specimen. In the equation for strain, L is the current length of the specimen and L0 is the original length.
The values of stress and strain determined from the tensile test can be plotted as a stress-strain curve, as shown below:
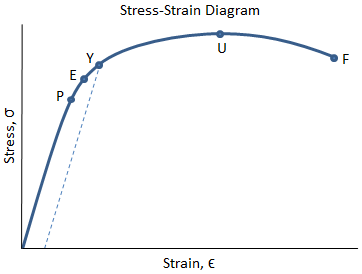
Check out our Stress-Strain Curve calculator based on the methodology described here.
There are several points of interest in the diagram above:
Stress-strain curves are commonly needed when analyzing an engineered component. However, stress-strain data may not always be readily available. In this case, it is fairly straightforward to approximate a material's stress-strain curve using the Ramberg-Osgood equation.
Engineers typically work with engineering stress, which is the force divided by the original area of the specimen before loading: σ = P/A0 . However, as a material is loaded, the area decreases. The true stress,
, is the value of stress in the material considering the actual area of the specimen. Because the area decreases as a material is loaded, true stress is higher than engineering stress.
The figure below shows an engineering stress-strain curve as compared to a true stress-strain curve. Because the engineering stress is calculated as force divided by original area (which is a constant), the engineering stress-strain curve has the same shape as the load-deflection curve. The engineering stress-strain curve drops after the ultimate strength is reached because the force that can be supported by the material drops as it begins to neck down. However, the stress value in the true stress-strain curve always increases as the strain increases. This is because the instantaneous value of area is used when calculating true stress. Even when the force supported by the material drops, the reduction in the specimen area outweighs the reduction in force, and the stress continues to increase.
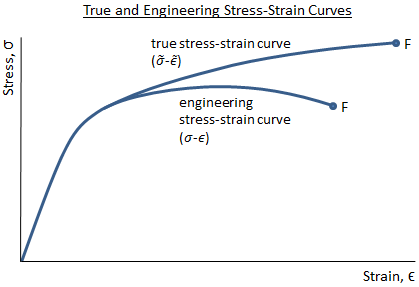
It should be noted that the engineering stress and the true stress are essentially the same in the linear-elastic region of the stress-strain curve. Because engineers typically operate within this linear-elastic region (it is uncommon to design a structure that is intended to operate beyond the elastic limit), it is valid to work with engineering stress as opposed to true stress.
Engineering strain is the change in length divided by the original length: ε = ΔL/L0 . Instead of just calculating a single value of ΔL , consider that the change in length is divided among many small increments, ΔLj . The strain is also calculated in small increments: εj = ΔLj/Lj , where ΔLj is the change in length for an increment, and Lj is the length at the start of the increment. As these increments become infinitesimally small, the summation of the strains approaches the true strain,
are the true stress and strain, and σ and ε are the engineering stress and strain.
Below the proportionality limit of the stress-strain curve, the relationship between stress and strain is linear. The slope of this linear portion of the stress-strain curve is the elastic modulus, E , also referred to as the Young's modulus and the modulus of elasticity. Hooke's law expresses the relationship between the elastic modulus, the stress, and the strain in a material within the linear region:
where σ is the value of stress and ε is the value of strain.
Hooke's law also has a form relating shear stresses and strains:
where τ is the value of shear stress, γ is the value of shear strain, and G is the shear modulus of elasticity. The elastic modulus and the shear modulus are related by:
The percent elongation is a commonly provided material property, so the plastic strain at failure is typically calculated from percent elongation:
εf = eL / 100%The ultimate strain accounts for both plastic and elastic strain at failure:
εu = εf + Stu/EAnother important material property that can be measured during a tensile test is the reduction in area, which is calculated by:
where σel and εel are the stress and strain at the elastic limit, Sty is the tensile yield strength, and E is the elastic modulus.
Note that the units of the modulus of resilience are the same as the units of strain energy density, which are psi in US Customary units and Pa in SI units.
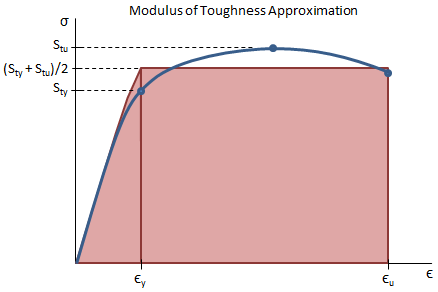
The modulus of toughness is the amount of strain energy per unit volume (i.e. strain energy density) that a material can absorb just before it fractures. The modulus of toughness is calculated as the area under the stress-strain curve up to the fracture point.
An accurate calculation of the total area under the stress-strain curve to determine the modulus of toughness is somewhat involved. However, a rough approximation can be made by dividing the stress-strain curve into a triangular section and a rectangular section, as seen in the figure below. The height of the sections is equal to the average of the yield strength and the ultimate strength.
The modulus of toughness can be approximated as:
Now that the constants n and H have been determined, the equation for the total strain as a function of stress is known:
The equation above can be simplified by substituting the expression for H . The final equation for total strain as a function of stress is:
PDH Classroom offers a continuing education course based on this mechanical properties of materials reference page. This course can be used to fulfill PDH credit requirements for maintaining your PE license.
Now that you've read this reference page, earn credit for it!